Question

The figure shows secants LH and and tangent K intersecting at point L. AHIL is a right triangle. Find mZHLJ, which isthe value for x, and m I, which is the value for yA. x = 274y = 86B. x = 137y = 43C. x=47y = 86D. x = 317y= 43

Answer 1

Given:

The figure shows secants LH and tangent K intersecting at point L. AHIL is a right triangle.

Required:

Find the value of x and y.

Step-by-step explanation:

The inscribed angle measures half of the arc it comprises.

`\begin{gathered} \angle GHL=(1)/(2)(arcLI) \\ 43\degree=(1)/(2)(arcLI) \\ Arc\text{ LI = 2}*43 \\ Arc\text{ LI = 86} \end{gathered}`
`\begin{gathered} \angle KLI=(1)/(2)(arc\text{ LI}) \\ \angle KLI=(1)/(2)(86) \\ =43\degree \end{gathered}`

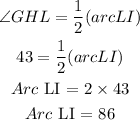
We know that the sum of linear angles is 180 degrees.

`\begin{gathered} \angle HLJ+\angle HLI+\angle ILK=180\degree \\ x+90\degree+43\degree=180\degree \\ x+133\degree=180\degree \\ x=180\degree-133\degree \\ x=47\degree \end{gathered}`

`\begin{gathered} x=47\degree \\ y=86 \end{gathered}`

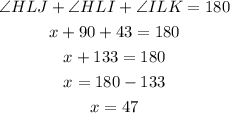
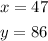
Final answer:

Option C is the correct answer.