Question

Which of the following sets of numbers could represent the three sides of a righttriangle?Submit Answer{11, 60, 62)O {45, 60, 74}O {51, 68, 86){33,56, 65)attempt 1 out of 2

Answer 1

To answer this question, we can use the Pythagorean Theorem to find three numbers that follow it. Then, we can proceed as follows:

1. We have that the hypotenuse has the greatest length in a right triangle. Then, we have:

First case: {11, 60, 62}

• Legs of the right triangle: 11, 60.

,

• Hypotenuse: 62

`11^2+60^2=121+3600=3721`

`62^2=3844`

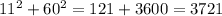
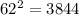
Therefore:

`11^2+60^2\\e62^2`

This set does not represent the three sides of a right triangle.

Second Case: {45, 60, 74}