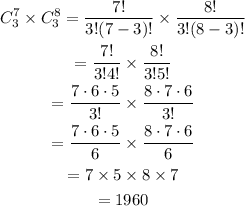We have to choose 3 teachers from a group of 7 teachers, then, the amount of combinations is given by
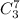
We have to choose 3 students from a group of 8 students, then, the amount of combinations is given by
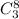
Since we have to choose a committee simultaneously formed by 3 teachers and 3 students, we want the intersection of those combinations, which is given by their product
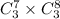
The formula for a combination is given by
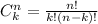
Then, we have