Let y = x^(4x). If we take the logarithm on both sides of the equation, we get the following:
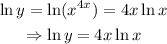
applying implicit derivation, we can find the derivative y':

therefore, f'(x) = 4x^(4x)(lnx + 1).
Then, for f'(1), we can make x = 1 on the derivative to get the following:
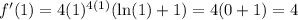
therefore f'(1) = 4