Question

Solve the following equation for X. Round your answer to four decimal places.

Answer 1

x = 1.2226

Step-by-step explanation

To solve this equation we have to apply the property of the logarithm of the base,

`\log _bb=1`

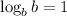
Thus, we can apply the natural logarithm - whose base is e, to both sides of the equation,

`\ln e^(4x)=\ln 133`

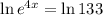
Now we apply the property of the logarithm of a power,

`\log a^b=b\log a`

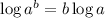
In our equation,

`\begin{gathered} 4x\ln e^{}=\ln 133 \\ 4x^{}=\ln 133 \end{gathered}`

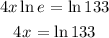
Then divide both sides by 4 and solve,

`\begin{gathered} \frac{4x^{}}{4}=(\ln 133)/(4) \\ x\approx1.2226 \end{gathered}`

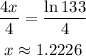
The solution to this equation is x = 1.2226, rounded to four decimal places.