Nancy: n
Olivia: o
Casandra: c
Nancy is four times as old as Olivia:

Olivia is three years less than Cassandra

The sum of their ages is 45:
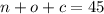
Use the next system of equations to solve How old is each girl?:
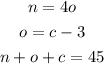
Write all the equation in terms of c:
Substitute the o in the first equation by the value of o in the second equation:

Substitite in the third equation the n and o by the corresponding values in terms of c:

Solve c:
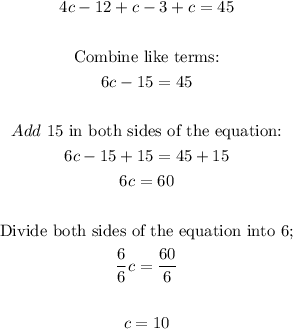
Use the value of c to solve the other two variables;
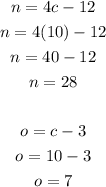
Then, Nancy is 28 years old, Olivia is 7 years old and Cassandra is 10 years old