Question

5. A bullet is fired horizontally from a 40 m tall buildingwith a speed of 1000 m/s. Calculate the vertical componentof its velocity just before it hits the ground? (1 point)A. O-8.804 m/sB. O-43.139 m/sC. O-11.785 m/sD. O-28 m/sE. O-51.282 m/s6. A bullet is fired horizontally from a 40 mtall building with a speed of 800 m/s.TT11.11 i 6-112 (1)itbeilePLESIREEFACITES)DETRISTESHEADSETromation

Answer 1

D. -28 m/s

Step-by-step explanation:

To find the final speed, we will use the following equation

`v_f^2=v_i^2+2g\Delta y`

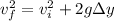
Where vf is the final velocity, vi is the initial vertical velocity, g is the acceleration due to gravity and Δy is the height.

Replacing vi = 0 m/s, g = 9.8 m/s², and Δy = 40 m, we get

`\begin{gathered} v_f^2=0^2+2(9.8)(40) \\ v_f^2=784 \\ v_f=√(784) \\ v_f=28 \end{gathered}`

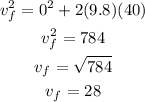
Therefore, the vertical component of the velocity is vf = -28 m/s because the bullet is going down.

So, the answer is

D. -28 m/s