Answer:
The scale factor of the smaller figure to the larger figure is 4:11 (option A)
Step-by-step explanation:
Given:
The volume of the smaller rectangular prism = 64 in^3
The volume of a larger rectangular prism = 1331 in^3
The prisms are similar
To find:
the scale factor of the smaller figure to the larger figure
For similar shapes, the scale factor of the shapes when the volumes are given:
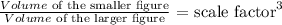
![\begin{gathered} (64)/(1331)\text{ = \lparen scale factor\rparen}^3 \\ \\ cube\text{ root both sides:} \\ \sqrt[3]{(64)/(1331)}\text{ = }\sqrt[3]{(scale\text{ factor\rparen}^3} \\ \\ \sqrt[3]{(4^3)/(11^3)}\text{ = }\sqrt[3]{(scale\text{ factor\rparen}^3} \\ \\ (4)/(11)\text{ = scale factor} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/high-school/lgdozirqjlmah6oytngf2rydfqr3jxfy93.png)
The scale factor of the smaller figure to the larger figure is 4:11 (option A)