1 Answer

Sandeep Dinesh · Answer 1 · 2023-06-15T16:16:06+0000

We must first assume that the base of the cone and the cylinder hvae tha same base shape and size.

The volumen of a cone is equal to one third of the volumen of the cylinder, whose base and height are equal those of the cone:

Therefore:

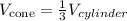
$V_{\text{cone}}=(1)/(3)V_(cylinder)$

replacing:

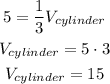
$\begin{gathered} 5=(1)/(3)V_(cylinder) \\ V_(cylinder)=5\cdot3 \\ V_(cylinder)=15 \end{gathered}$

The answer is 15 in^3

Please log in or register to add a comment.