Given data
*The given wavelength of the ultraviolet light is
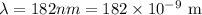
*The given total energy is E_t = 1.28 × 10^-13 J
The formula for the difference in energy between the two levels that participate in stimulated emission in the excimer laser is given as
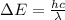
*Here h = 6.626 × 10^-34 J.s is the Planck's constant.
*Here c = 3.0 × 10^8 m/s is the speed of the light.
Substitute the known values in the above expression as
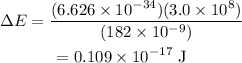
Hence, the difference in energy between the two levels that participate in stimulated emission in the excimer laser is 0.109 × 10^-17 J
The number of photons from this laser is required to deliver energy is calculated as
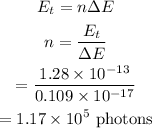
Hence, the number of photons from this laser is required to deliver energy is n = 1.17 × 10^5 photons