Remember that
the distance between two points is equal to
![d=\sqrt[\square]{(y2-y1)^2+(x2-x1)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/t42uwhq5jpr9srsdp5ljh323ya0c3721co.png)
In this problem we have
d=6 units
A(-2,5)
B(x,y)
substitute the given values in the expression above
![6=\sqrt[\square]{(y-5)^2+(x+2)^2}](https://img.qammunity.org/2023/formulas/mathematics/high-school/m4d40ure7ke24gjfvkmc8qukdcizycuh0s.png)
squared both sides

Find out three possible coordinates of point B
First and Second possibles coordinate
I will assume the x-coordinate
For x=-2
substitute in the expression above and solve for y
36=(y-5)^2
square root both sides

Values of y are
y=11 and y=-1
therefore
we have the coordinates of point B
(-2,11) and (-2,-1)
Find out the third possible coordinate of point B
I will assume the y-coordinate
For y=5
36=(x+2)^2
square root both sides
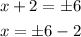
the values of x are
x=4 and x=-8
the possibles values of B are
(4,5) and (-8,5)
therefore
Possibles values of point B are
(-2,11)
(-2,-1)
(4,5)
(-8,5)
Part 2
Find out the midpoint of each of those possible segments
The formula to calculate the midpoint between two points is equal to

so
For A(-2,5) and B(-2,11)
substitute in the formula-------> M(-2,8)
For A(-2,5) and B(-2,-1) ------> M(-2,2)
For A(-2,5) and B(4,5) -------> M(1,5)
For A(-2,5) and B(-8,5) -----> M(-5,5)