Question

The cost in dollars of making x items is given by the function C(x)=10x+500. a. The fixed cost is determined when zero items are produced. Find the fixed cost for this item. Fixed cost =$ b. What is the cost of making 25 items? C(25)=$ c. Suppose the maximum cost allowed is $2500. What are the domain and range of the cost function, C(x)? When you enter a number in your answer, do not enter any commas in that number. In other words if you want to enter one thousand, then type in 1000 and not 1,000. It's not possible to understand what the interval (1,000,2,000) means, so you should write that as (1000,2000). Domain: Range:

Answer 1

We have the cost of making x items with t he function:

`C(x)=10x+500`

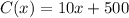
a) The fixed cost is equivalent to C(0), so we calculate it as:

`x=0\Rightarrow C(0)=10\cdot0+500=500`

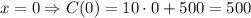
The fixed cost is $500.

b) The cost of making 25 items can be calculated as C(25):

`\begin{gathered} C(25)=10\cdot25+500 \\ C(25)=250+500 \\ C(25)=750 \end{gathered}`

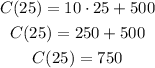
C(25) = $750

c) If the maximum cost is $2500 and the minimum cost is $500 (as we can not manufacture less than 0 items and this is the fixed cost), the range can be defined as:

Range: [500, 2500]

The domain has a lower bound at x = 0, as there is no cost function for a negative amount of items.

The greatest number of items for this function will match the greatest cost, that is $2500.

So we can calculate the maximum value for x as:

`\begin{gathered} C(x)=2500 \\ 10x+500=2500 \\ 10x=2500-500 \\ 10x=2000 \\ x=(2000)/(10) \\ x=200 \end{gathered}`

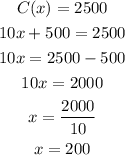
Then, C(x) will be defined for x between 0 and 200. The domain is [0, 200].

Answer:

a) The fixed cost is $500.

b) C(25) = $750

c) Domain: [0, 200]

Range: [500, 2500]