Question

1. Match each graph with a function given at right. Explain your process for making the matches.

Answer 1

The first graph is given as

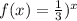
`\begin{gathered} f\mleft(x\mright)=3^x \\ when\text{ x=0} \\ y=3^x \\ y=3^0 \\ y=1 \\ \left(0,1\right) \\ when\text{ x=1} \\ y=3^1 \\ y=3 \\ \left(1,3\right) \end{gathered}`

Hence,

The graph is given below as

The second equation is given below as

`f\mleft(x\mright)=\lparen(1)/(3))^x`

Hence,

The graph is given below as

The third function is given below as

`\begin{gathered} f\mleft(x\mright)=\left((2)/(3)\right?^x \\ when\text{ x=0} \\ y=\left((2)/(3)\right?^0 \\ y=1 \\ \left(0,1\right) \\ \\ when\text{ x=-1} \\ y=\left((2)/(3)\right?^(-1) \\ y=(3)/(2)=1.5 \\ \left(-1,1.5\right) \end{gathered}`

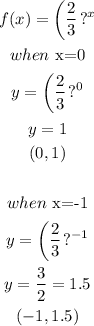
The graph is given below as

The fourth equation is given below

`\begin{gathered} f\mleft(x\mright)=4^x \\ when\text{ x=0} \\ y=4^0=1 \\ \left(0,1\right) \\ \\ When\text{ x=1} \\ y=4^1=4 \\ \left(1,4\right) \end{gathered}`

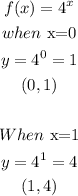
The graph is given below as

Hence,

The final answer is given in the image below as