We have that the local maximum is 282 at x = 4, this means that this function has a local maximum at x = 4 with output value 282 because
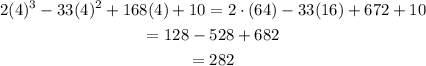
this function has a local minimum at x= 7 with output value 255
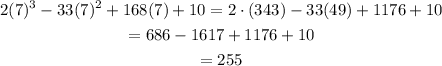
We have that the graph is not easy to see in any scale