Answer:
The scale factor is 2.
Step-by-step explanation:
To determine the scale factor using the coordinates of each point, simply divide the respective coordinates of the new figure by the old figure.
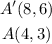
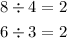

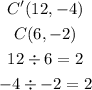
As we can see above, the ratio of the coordinates of the new image triangle A'B'C and the original image triangle ABC is 2. Hence, the scale factor is 2.