Answer:
0.96 = 96% probability that at least one of them detect an enemy attack.
Explanation:
For each radar, there are only two possible outcomes. Either it detects the attack, or it does not. The missiles are operated independently, which means that we use the binomial probability distribution to solve this question.
Binomial probability distribution
The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.
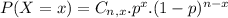
In which
 is the number of different combinations of x objects from a set of n elements, given by the following formula.
is the number of different combinations of x objects from a set of n elements, given by the following formula.
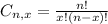
And p is the probability of X happening.
Assume that a particular detection system has a 0.80 probability of detecting a missile attack.
This means that

If two military radars are installed in two different areas and they operate independently, the probability that at least one of them detect an enemy attack is
This is
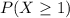 when
when
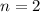 . So
. So

In which
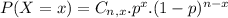
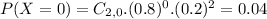
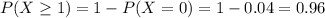
0.96 = 96% probability that at least one of them detect an enemy attack.