Question

investment A: 4,000 invested for 7 years compounded semiannually at 8%investment b: 6,000 invested for 4 years compounded quarterly at 3.6%

Answer 1

Investment A: 4000 invested for 7 years compounded semi annually at 8%

Let the principal = $4,000

rate = 8%

time = 7 years

n = 2 because it is compounded semi anually

`\begin{gathered} \text{Compound interest formula is written as} \\ A\text{ = P( 1 + }(r)/(n))^{n\text{ x t}} \\ P\text{ = \$4, 000} \\ r\text{ = 8\% = 0.08} \\ t=\text{ 7} \\ n\text{ = 2} \\ A\text{ = 4000( 1 + }(0.08)/(2))^{7\text{ x 2}} \\ A=4000(1+0.04)^(14) \\ A=4000(1.04)^(14) \\ A\text{ = 4000 x 1.7317} \\ A\text{ = \$6, 926.71} \end{gathered}`

The compound interest for investment A for 7 years is $6, 926.71

For investment B

The amount invested is $6000 for 4 years and its compounded quarterly at 3.6%

The compound interest formula is written as

`\begin{gathered} A\text{ = P( 1 + }(r)/(n))^{n\text{ x t}} \\ P\text{ = \$6000} \\ r\text{ = 3.6\% = 0.036} \\ n\text{ = 4 because it is compounded quartely} \\ t\text{ = 4} \\ A\text{ = 6000( 1 + }(0.036)/(4))^{4\text{ x 4}} \\ A=6000(1+0.009)^(16) \\ A=6000(1.009)^(16) \\ A\text{ = 6000 x 1.154} \\ A\text{ = \$6, 924.84} \end{gathered}`

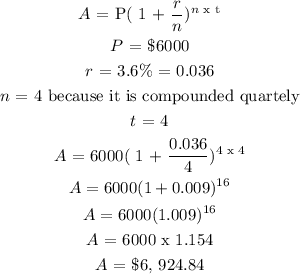
The compound interest for investment B for 4 years is $6, 924.48