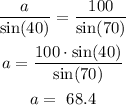Question

20.Solve the triangle: b = 100, c = 100, y = 70". If it is not possible, say so.This triangle is not solvable.Q = 40", a = 68.4, B = 70'a= 70', a = 68.4, B = 40"Q = 40", a = 100, B=70"

Answer 1

`\begin{gathered} \alpha=\text{ 40\degree} \\ \beta=\text{ 70\degree} \\ a=68.4 \end{gathered}`

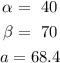
Explanation:

By the theorem of intern angles of a triangle, we know that the sum of all intern angles of a triangle must be 180°, therefore:

*There are two equal angles because there are two equal sides

`\begin{gathered} \alpha=180-70-70 \\ \alpha=\text{ 40\degree} \\ \beta=\text{ 70\degree} \end{gathered}`

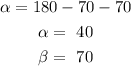
Now, applying the law of sines, we can find side a. The law of sines is represented by the following equation:

`(a)/(\sin \alpha)=(b)/(\sin \beta)`

Hence, if b=100, alpha=40° and betha=70°, solve for a:

`\begin{gathered} (a)/(\sin (40))=(100)/(\sin (70)) \\ a=(100\cdot\sin (40))/(\sin (70)) \\ a=\text{ 68.4} \end{gathered}`