Given the model equation of the first question:
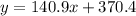
And assuming "x" stands for the time, "t", in days, we can calculate which day, "x", the number of infected people will reach 7000 by setting y = 7000 and solving for x:

So, the number of infected people will reach 7000 around day 47.