In order to calculate the angle of the refracted ray, we can use the law of refraction (Snell's Law) below:
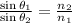
Where n1 and n2 are the indexes of refraction and theta1 and theta2 are the angles of the ray.
So, using n1 = 1, n2 = 1.65 and theta1 = 30°, we have:
