Question

a cube shaped dog kennel is replaced by a larger kennel the volume of the original kennel was 27 cubic feet the volume of the new kennel is 64 cubic feet how many feet were added to eat Edge length of the kennel 1 foot 2 ft 3 ft 4 ft

Answer 1

The volume V of a cube is given by

`V=L^3`

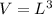
where L is the lenght of one side. Hence, the side L measures

`L=\sqrt[3]{V}`

In the first case, the dog kennel has 27 ft^3 of volume, hence

`\begin{gathered} L=\sqrt[3]{27} \\ L=3\text{ } \end{gathered}`

that is, L is 3 feets of lenght.

In the same way, for the second case we have that,

`\begin{gathered} L=\sqrt[3]{64} \\ L=4 \end{gathered}`

that is, in the second case, L is 4 feet of lenght. This means that, 1 foot was added to each edge of the dog kennel.