Question

4A. List the transformations needed to move the graph of =2so that it lies exactly on top of the graph of =−32(+5)2−1 Be specific: for example you could say, move the graph up 3 units or vertically stretch the graph by a factor of 5.B. Sketch the transformed graph. Be to label the axes and the scale used.

Answer 1

We are required to move the graph

`y=x^2\text{ }`

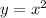
so that it lies on top of the graph

`y=-(3)/(2)(x+5)^2-1`

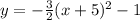
The original graph and the transformed graph are shown below:

The original graph is represented by the red graph

`y=x^2`

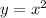
The transformed graph is represented by the function

`-(3)/(2)(x+5)^2-1`

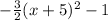
The transformation rule is:

1. Reflect it on the x-axis

2. Bring the graph down vertically by 1 unit

3. Shift the graph 5 units to left

4. Stretch the graph by a factor of 3/2