Cynthia can install 50 shingles per hour
Here, we want to get the number of shingles Cynthia can install per hour
From the question, we have it that for the same one hour, Timothy install more shingles
Let the rate taken for Cynthia be x shingles per hour
Since Timothy installs 10 more, then the rate for Timothy will be (10 + x) shingles per hour
Since their hourly rate is equal, we have it that;
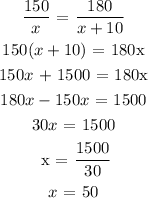
What this mean is that Cynthia fixes 50 shingles per hour