The coordinates of the vertices of the original figure are
A(5, 0), B(0, 5), C(-5, 0), and D(0, -5)
Therefore OB = 5, and OA = 5
Since AOB is a right-angled triangle with hypotenuse AB,
then
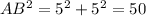
Hence,
![AB=5\sqrt[]{2}](https://img.qammunity.org/2023/formulas/mathematics/college/ah8eown8o8gnn6ha5o06axg5bid1dymp93.png)
From the graph, we can see that
BD = 10 =AC
and AC is perpendicular to BD
Therefore the figure is a square,
Which means that
![AB=BC=CD=DA=5\sqrt[]{2}](https://img.qammunity.org/2023/formulas/mathematics/college/u92t05jf66l21za3p6kooo1rjd4j21wdog.png)
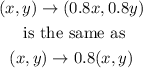
Hence the image of the figure under the transformation is also a square with the length of a side equal to the product of 0.8 and the length of side of a side of the original figure
That is
![\text{ length of each side of image =0.8}*5\sqrt[]{2}=4\sqrt[]{2}](https://img.qammunity.org/2023/formulas/mathematics/college/c4lac7rd64gfjz9k3zv7vwe0hjnnxpa0lw.png)
Therefore
![\begin{gathered} A^(\prime)B^(\prime)\text{ = 4}\sqrt[]{2} \\ B^(\prime)C^(\prime)\text{ = 4}\sqrt[]{2} \\ C^(\prime)D^(\prime)\text{ = 4}\sqrt[]{2} \\ D^(\prime)A^(\prime)\text{ = 4}\sqrt[]{2} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/cbr85c3oveqk3op16pgtgl1w9150b28gp2.png)