Question

50.0 mL of an acetic acid (CH3COOH) solution is titrated with 0.100 M NaOH solution; you reach the equivalence point, as judged by your acid-baseindicator, when 36.4 mL of the NaOH solution has been added. What is the concentration of the acetic acid solution?O 0.728 MO 0.100 MO 0.0728 MO 7.28 x 10 5M

Answer 1

The question requires us to calculate the molar concentration of an acetic acid solution, given that it was necessary 36.4 mL of a 0.100 M NaOH solution to neutralize 50.0 mL of the acid.

The following information was provided by the question:

concentration of NaOH solution = C(OH-) = 0.100 M

volume of NaOH solution = V(OH-) = 36.4 mL

volume of CH3COOH solution = V(H+) = 50.0 mL

Acetic acid (CH3COOH) and sodium hydroxide (NaOH) react according to the following reaction:

`\text{CH}_3COOH_{}+NaOH_{}\to CH_3COONa+H_2O`

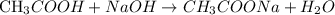
Considering that at the equivalent point the number of moles of acid (H+) is the same as the number of moles of base (OH-) and taking the stoichiometry of the reaction into consideratio (1 : 1), we can write:

`At\text{ equivalent point}\to n_(H^+)n_(OH^-)\to n_(CH_3COOH)=n_(NaOH)`

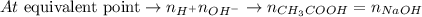
We can also write the number of moles of a compound (n) in terms of its molar concentration and volume:

`\begin{gathered} \text{molar concentration = }\frac{number\text{ of moles}}{\text{volume}}\to C=(n)/(V) \\ \\ n=C* V \end{gathered}`

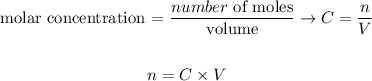
Thus, considering the equivalent point, we can say:

`n_(CH_3COOH)=n_(NaOH)\to C_(CH_3COOH)* V_(CH_3COOH)=C_(NaOH)* V_(NaOH)`

Now, we can apply the values of volume and concentration provided by the question to the equation above and obtain the concentration of the acid:

`\begin{gathered} C_(CH_3COOH)* V_(CH_3COOH)=C_(NaOH)* V_(NaOH) \\ C_(CH_3COOH)*(50.0mL)=(0.100M)*(36.4mL) \\ C_(CH_3COOH)=((0.100M)*(36.4mL))/((50.0mL))=0.0728M \end{gathered}`

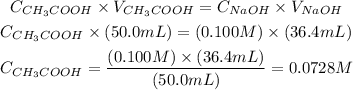
Therefore, the molar concentration of the acetic acid solution is 0.0728 M and the best option to answer this question is the third one.