WU = 30.2 ft
Here, we want to find the side marked x to the nearest foot
From the question, we need to get the sides of the triangle given
The side facing the right-angle is the longest side and it is called the hypotenuse
The side that faces the angle given is known as the opposite
With respect to the question, the hypotenuse is marked 40, while the opposite is marked x
Mathematically, we will need a trigonometric identity that links both
The trigonometric identity that links the hypotenuse and the opposite is the sine
The sine of an angle is the ratio of the length of thr opposite to that of the hypotenuse
We have this as;
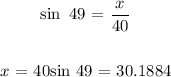
To the nearest tenth of a foot, this is 30.2 foot