Answer:
Remainder = 0
Step-by-step explanation:
The Remainder theorem says that when we divide a function f(x) by a function with the form (x - c), the remainder will be equal to f(c)
Therefore, we can rewrite the expression as:
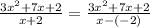
So, we can say that f(x) = 3x² + 7x + 2 and (x - c) = (x -(-2)). Then by the Remainder theorem, the remainder will be:
f(c) = f(-2)
So, f(-2) is equal to:
f(x) = 3x² + 7x + 2
f(-2) = 3(-2)² + 7(-2) + 2
f(-2) = 3(4) - 14 + 2
f(-2) = 12 - 14 + 2
f(-2) = 0
Therefore, the remainder will be 0.