The first thing to know is that the probability of drawing any card is

Now within a deck of 52 cards we have 12 figures in total. Also the deck can be either black or red.
A) So for the first part the probability that from a deck of 52 cards you will draw a face card is:
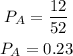
B) And if we want to know if the letter that we will draw is only black:
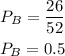
C) If we want to know what is the probability that we will draw from a deck a card that is face card and black, we have to know how many cards are black of the 12 that are face card, as we only have 2 options or red or black the number of black figure cards are 6
