Question

If f(x) is defined as follows, find (a) , (b) , and (c) .

Answer 1

We have a piecewise function f(x). This means it has different definitions for different intervals of x.

In this case we want to know the value of f(-1), which is the value of the function when x=-1.

Then, we have to look where x=-1 is located in the definition of f(x).

If we look at the definition of f(x), x=-1 falls in the first part, where f(x) is defined for x<0. Then, f(x) = x² for x=-1:

`\begin{gathered} f(x)=x^2 \\ f(-1)=(-1)^2=1 \end{gathered}`

If we want to calculate f(0), we should look where x=0 is located in the definition of f(x).

In this case, f(x) = 1 for x=0.

Then:

`\begin{gathered} f(x)=1 \\ f(0)=1 \end{gathered}`

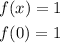
Answer:

f(-1) = 1

f(0) = 1