1) Let's begin with that, by using the Rational Zero Theorem
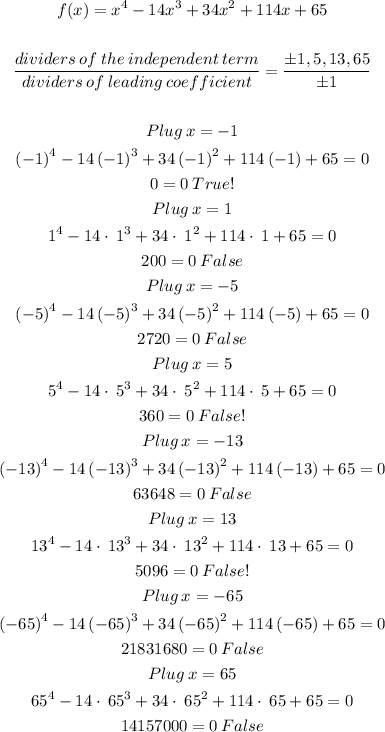
So, with the Rational Zero Theorem method, we could only find one root.
x=-1
2)Now, let's apply the Descartes' Rule of Signs
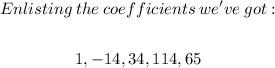
Note that there are two sign changes, so there are two or no
positive roots