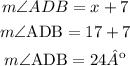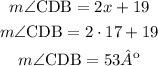To solve this exercise you have to use the angle addition postulate that states that the sum of the measures of two adjacent angles (that share the same vertex) is equal to the measure of the angle they form.
With this in mind, we can determine that
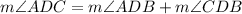
If
m∠ADC= 77º
m∠ADB= (x+7)º
m∠CDB= (2x+19)º
We can determine the following equation
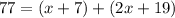
From this expression, we can determine the value of x, first step is to remove the parentheses and simplify the like terms:
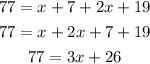
Next, pass "+26" to the other side of the equation by applying the opposite operation, i.e. subtract it from both sides of the equal sign
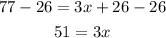
Finally, divide both sides by 3 to determine the value of x
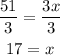
Once calculated the value of x, you can calculate the measures of both angles