ANSWER
12.45 moles of H2SO4 will reacts completely with 8.3 mol of Al
Step-by-step explanation
Given information
The number of moles of Al is 8.3 mol
To find the number of moles of H2SO4 that reacts with Al, you will need to follow the steps below
Step 1: Write a balanced equation between the reaction Al and H2SO4
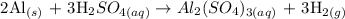
The aim of the above reaction is to produce hydrogen gas. From the above reaction, you will see that 2 moles of aluminum react with 2 moles of H2SO4.
Since we already know the number of moles of Al, therefore, we can calculate the number of moles of H2SO4 using a stoichiometric ratio
Step 2: Find the number of moles of H2SO4
Let x represents the number of moles of H2SO4
According to their stoichiometric ratio; 2 moles of Al react with 3 moles of H2SO4
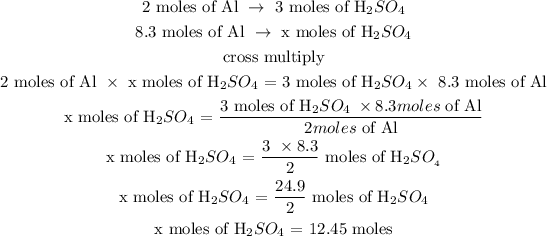
Hence, 12.45 moles of H2SO4 will reacts completely with 8.3 mol of Al