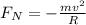Question

What is the magnitude of a force acting in the outward radial direction acting on a particle traveling in a circle?

Answer 1

mv^2 / R

Step-by-step explanation:

If a particle of mass m is going in a circle of radius R with velocity v, then the centripetal force is

`F=(mv^2)/(R)`

Now, the force that counters centripetal force is the normal force ( which is the result of Newton's third law - every force comes with an equal and opposite force) and its magnitude is exactly to that of the centripetal force.

`F_N=-(mv^2)/(R)`