Question

What is the surface area of the solid figure represented by the net? 94 cm 127 cm 13.9 cm 5.6 cm -

Answer 1

For this problem, we are provided with all the faces of a solid figure, as well as its measurements. We need to determine the surface area of the image.

Let's draw the image and identify all the faces:

The total surface area will be equal to the sum of the area of each face. We have:

`\begin{gathered} A_1=length*width=9.4\cdot5.6=52.64\text{ square cm}\\ \\ A_2=length*width=12.7\cdot9.4=119.38\text{ square cm}\\ \\ A_3=length*width=13.9\cdot9.4=130.66\text{ square cm}\\ \\ A_4=A_5=(base*height)/(2)=(5.6\cdot12.7)/(2)=35.56\text{ square cm} \end{gathered}`

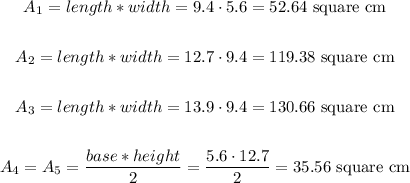
Now we need to sum all the areas:

`\begin{gathered} A=A_1+A_2+A_3+A_4+A_5 \\ A=52.64+119.38+130.66+35.56+35.56=373.8\text{ square cm} \end{gathered}`

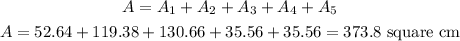
The surface area is equal to 373.8 square centimeters.