Given:
The perimeter of the rectangle is 28 units.
To create:
The table shows the length and width of at least 3 different rectangles that also have a perimeter of 28 units.
Step-by-step explanation:
Let x be the length of the rectangle.
Let y be the width of the rectangle.
Then the perimeter of the rectangle is,
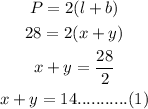
When x = 1, we get y = 13.
When x = 2, we get y = 12.
When x = 3, we get y = 11.
When x = 4, we get y = 10.
So, the table values are,
The relationship between the columns is,
When x increases by 1 unit, then y decreases by 1 unit.
The expression is,
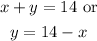
Here, x is the independent variable and y is the dependent variable.
The graph is,