Question

Find the angle between the forces given the magnitude of their resultant. (Hint: Write force 1 as a vector in the direction of the positive x-axis and force 2 as a vector at an angle with the positive x-axis. Round your answer to one decimal place.)

Answer 1

First, let's draw the vectors and the resultant vector:

In order to calculate angle theta, first let's find the angle created between the Force 1 in black and the Force 2 in purple. Then, angle theta will be supplementary to this angle, since the figure created is a parallelogram.

To find the angle above, we can use the law of cosines in the bottom triangle:

`\begin{gathered} R^2=F_1^2+F_2^2-2F_1F_2\cos(x)\\ \\ 105^2=55^2+70^2-2\cdot55\cdot70\cdot\cos x\\ \\ 11025=3025+4900-7700\cos x\\ \\ 7700\cos x=-3100\\ \\ \cos x=-0.402597\\ \\ x=113.74° \end{gathered}`

Now, let's calculate angle theta:

`\begin{gathered} \theta+113.74=180\\ \\ \theta=180-113.74\\ \\ \theta=66.26° \end{gathered}`

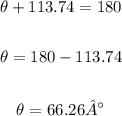
Rounding to one decimal place, we have theta equal to 66.3°.