Question

....................

Answer 1

The Equation of a Line

The slope-intercept of the line is:

y = mx + b

Where m is the slope and m is the y-intercept of the line.

The point-slope form of the line is:

y = m(x - h) + k

Where (h, k) is a point of the line.

We know our line is parallel to:

6x + y = 6

Parallel lines have the same slope. The slope of this line can be found by solving for y:

`y=-6x+6`

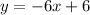
The slope of the given line and the slope of our required line is m = -6.

We use the point-slope form with the slope m = -6 and the point (2, -5):

`\begin{gathered} y=-6(x-2)-5 \\ \text{Operating:} \\ y=-6x+12-5 \\ y=-6x+7 \end{gathered}`

The required equation of the line is:

y = -6x + 7