Question

Match each exponential equation to its percent rate of change. Not all tiles will be used

Answer 1

Step-by-step explanation

In general, an exponential equation has the form

`a\cdot b^t=c,`

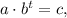
where a, b, and c are constants. The percent rate of change of such an equation is given by

`b-1.`

If this number is positive, we talk about growth; but if this number is negative, we talk about decay.

With this in mind, let's solve the exercise:

• The percent rate of change of the first equation is

`1.6-1=0.6;`

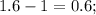
which in percentage form turns out to be

`0.6\cdot100=60\%.`

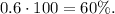
• The percent of change of the second equation is

`1.4-1=0.4;`

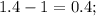
which in percentage form turns out to be

`0.4\cdot100=40\%\text{.}`

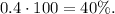
• The percent rate of change of the third equation is

`0.8-1=-0.2.`

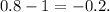
Which in percentage form turns out to be

`-0.2\cdot100=-20\%\text{.}`

• The percent rate of change of the fourth equation is

`0.2-1=-0.8;`

which is percentage form is

`-0.8\cdot100=-80\%\text{.}`

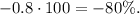
• The percent rate of change of the last equation is

`0.6-1=-0.4;`

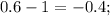
which in percentage form is

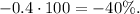
`-0.4\cdot100=-40\%\text{.}`Answer