According to the given sequence, its difference is +2, the first element is 1, and the problem is asking for the number of terms between 315 and 639.
First, we have to find the position of 315 and 639, in other words, we have to find n for each case.
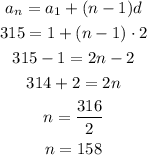
Hence, the term 315 is the 158th.
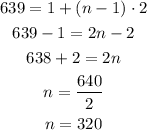
Hence, the term 639 is the 320th.
Then, we subtract the positions.
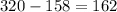
Therefore, there are 162 terms between 315 and 639.