Question

Given the figure below, find the values of x and z. (9x - 17) (12x - 62)

Answer 1

The diagram shows two pairs of angles lying on a straight line. Please remeber that angles on a straight line equals 180. Also you can observe two pairs of vertically opposite angles, and vertically opposite angles are equal. Hence, the angle opposite angle z also equals z. This means angles 9x - 17 and z (to the right) lie on a straight line. Similarly angle 12x - 62 and z (to the left) also lie on a straight line. Therefore;

`\begin{gathered} 9x-17+z=180\text{ -----(1)} \\ 12x-62+z=180\text{ ----(2)} \\ \text{Fro equation (1), make z the subject and you have} \\ z=180-9x+17 \\ z=197-9x \\ \text{Substitute for the value of z into equation (2)} \\ 12x-62+197-9x=180 \\ 12x-9x+197-62=180 \\ 3x+135=180 \\ \text{Subtract 135 from both sides} \\ 3x=45 \\ \text{Divide both sides by 3} \\ x=15 \\ \text{Substitute for the value of x into equation (1)} \\ 9x-17+z=180 \\ 9(15)-17+z=180 \\ 135-17+z=180 \\ 118+z=180 \\ \text{Subtract 118 from both sides} \\ z=62 \end{gathered}`

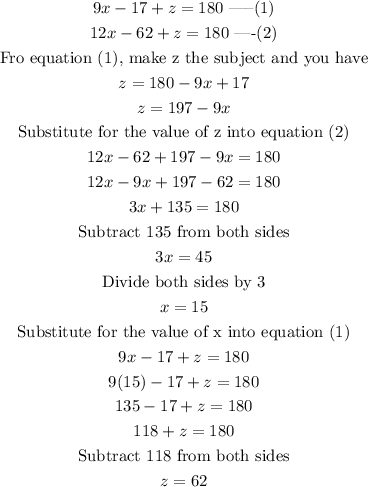
Therefore, x = 15 and z = 62