Answer:
C) -3 < x < 1
Explanation:
Given the absolute inequality:
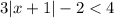
First, add 2 to both sides of the equation:
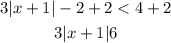
Next, divide both sides by 3:
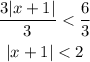
We then solve the absolute inequality:
[tex]\begin{gathered} -2
The solution to the absolute inequality is -3Option C is correct.