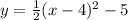To find the equation of the parabola knowing the vertex (4,-5) and a point (-6,45) you have to use the vertex form:

Replace the formula with:
Vertex:
h=4
k=-5
Point
x=-6
y=45
To calculate a
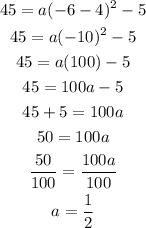
Now that we know the value of a we can write the equation of the parabola as: