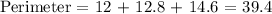Question

Find the perimeter of the triangle: ROUND TO YOUR ANSWER TO THE NEAREST TENTH. To find perimeter you must add up all of the sides. 12 10 8

Answer 1

The perimeter of the triangle to the nearest tenth is 39.4 units

Here, we want to find the perimeter of the triangle

To do this, we need the measures of the three sides

As it stands in the image, we only have the measure of one side completely

The triangle is divided into 2 right-triangles

We can use the Pythagoras' theorem to find the missing lengths

We start with the one on the left. We need the measure of its base

Pythagoras' theorem states that the square of the hypotenuse equals the sum of the squares of the two other sides. The hypotenuse is the longest side and it faces the right-angle

Let the missing length (base) of the right-triangle on the left be x

Mathematically;

`\begin{gathered} 10^2+x^2=12^2 \\ 100+x^2\text{ = 144} \\ x^2\text{ = 144-100} \\ x^2\text{ = 44} \\ x\text{ = }\sqrt[]{44} \\ x\text{ =6.6} \end{gathered}`

To get the total base length of the base, we add what we have here to 8

That would give the entire base length of the whole triangle as;

`6.6\text{ + 8 = 14.6}`

Furthermore, we need the length of the third side. This is the hypotenuse for the right-triangle on the left. Let us label this side as y

We have this as;

`\begin{gathered} y^2=10^2+8^2 \\ y^2\text{ = 100 + 64} \\ y^2\text{ = 164} \\ y\text{ = }\sqrt[]{164} \\ y\text{ = 12.8} \end{gathered}`

Now, we can calculate the perimeter as follows;

`\text{Perimeter = 12 + 12.8 + 14.6 = 39.4}`