Question

A billiard ball with a speed of 5 m/s strikes another stationary billiard ball in a perfectly elastic collision.

After the collision, the first ball has a speed of 4.35 m/s and is traveling at an angle of 30° below its original line of motion.

Find the speed and angle of the second ball, with respect to the initial line of motion, after the collision.

Answer 1

Assuming both billiard balls have the same mass, conservation of momentum says

`m\vec v_1 + m\vec v_2 = m{\vec v_1}\,' + m{\vec v_2}\,'`

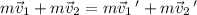
where m = mass of both billiard balls, and v₁ and v₂ = their initial velocities, and v₁' and v₂' = their final velocities. The masses are the same so the exact value of m is irrelevant. The first ball has initial speed 5 m/s and the second is at rest, so

`\left(5 (\rm m)/(\rm s)\right) \, \vec\imath = {\vec v_1}\,' + {\vec v_2}\,'`

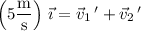
After the collision, the first ball has speed 4.35 m/s and is moving at angle of 30° below the original path, so

`{\vec v_1}\,' = \left(4.35(\rm m)/(\rm s)\right)\left(\cos(30^\circ) \, \vec\imath + \sin(30^\circ) \, \vec\jmath\right) \approx \left(3.77 (\rm m)/(\rm s)\right) \vec\imath + \left(-2.18 (\rm m)/(\rm s)\right) \vec\jmath`

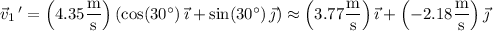
Then the second ball has final velocity vector

`{\vec v_2}\,' \approx \left(1.23 (\rm m)/(\rm s)\right) \vec\imath + \left(2.18 (\rm m)/(\rm s)\right) \vec\jmath`

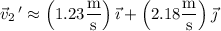
so it moves with speed

`\left\|{\vec v_2}\,'\right\| \approx \sqrt{\left(1.23(\rm m)/(\rm s)\right)^2 + \left(2.18(\rm m)/(\rm s)\right)^2} \approx \boxed{2.50 (\rm m)/(\rm s)}`

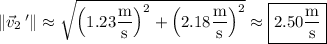
at an angle of

`\theta \approx \tan^(-1)\left((2.18)/(1.23)\right) \approx \boxed{60.5^\circ}`

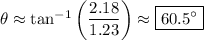
or about 60.5° above the original line of motion.