Hello there. To solve this question, we have to remember some properties about exponential functions.
Given the following function:
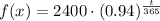
We want to determine whether it is
growing or decaying exponentially
at which rate and
for which unit of time
In this case, notice it has the following form:
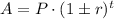
For this function, P is the principal value, r is the interest rate and t is the time period with the same units as the interest rate.
The plus minus sign stands for whether it is growing or decaying and, of course, r is greater than zero and usually less than 1.
We can rewrite our expression in the following way
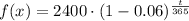
So we already know that r = 0.06 or 6% and the minus sign tells us that it is decaying exponentially.
The time unit has to do with the periodization and it can be yearly, monthly, daily...
Usually, it is divided by the factor for which it will turn from years to the desired periodization. In our case, it is divided by 365 and we know that a year has 365 days.
Hence we say that the final answer is:
The function is decaying exponentially at a rate of 6% every day.