Question

) Find the image of P(-5,2) after two transformations; first reflection over the y axis and thenrotated 90 counter clockwise about the origin.P'P”

Answer 1

`\begin{gathered} P^(\prime)(5,2) \\ P^(\prime\prime)\text{ (-2,5)} \end{gathered}`

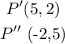
Step-by-step explanation

Step 1

The rule for reflecting over the Y axis is to negate the value of the x-coordinate of each point, but leave the y-value the same

`A(x,y)\rightarrow A^(\prime)(-x,y)`

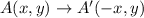
then

`P(-5,2)\rightarrow P^(\prime)(-(-5).2)\rightarrow P^(\prime)(5,2)`

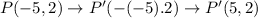
Step 2

then

rotated 90 counter clockwise about the origin.

When rotating a point 90 degrees counterclockwise about the origin our point P(x,y) becomes P'(-y,x). In other words, switch x and y and make y negative

`\begin{gathered} P(x,y)\rightarrow P(-y,x) \\ \end{gathered}`

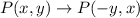
then

`\begin{gathered} P^(\prime)(5,2)\rightarrow P^(\prime)^(\prime)\text{ (-2,5)} \\ P^(\prime\prime)\text{ (-2,5)} \end{gathered}`

I hope this helps you