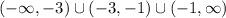Solution
- The domain is simply all the possible x-values for which the rational function is defined.
- In order for us to find the domain, we simply need to find the vertical asymptotes of the function and then exclude them from the domain.
- The vertical asymptotes of a rational function is simply all the values of x, or in this case, t, that make the function undefined.
- Thus, we should equate the denominator to zero and then find the values of t. These values of t will be the values to exclude from the domain.
- Thus, we have:

- Thus, the values to exclude from the domain are t = -1 and t = -3.
- Thus, the domain of the function is