Question

A 2 mg sample of Au-198, which is sometimes used in medicine, has a half life of 2.7 days. Write an equation and then find the amount remaining after 5 days.Equation:Answer:

Answer 1

Answer:

The amount of Au-198 remaining after 5days is 0.554mg

Step-by-step explanation:

Given:

initial amount of Au-198= 2mg

half-life = 2.7 days

To find:

Amount remaining after 5 days

To determine the amount remaining, we will apply the half-life formula:

`\begin{gathered} N(t)\text{ = N}_0((1)/(2))^{(t)/((t1)/(2))} \\ where\text{ N\lparen t\rparen = amount remaining} \\ t\text{ = time} \\ t_{(1)/(2)}=\text{ half-life} \end{gathered}`

We will write the equation in terms of t. Substitute other values into the formula except the value of t:

`\begin{gathered} N(t)\text{ = 2\lparen}(1)/(2))^{(t)/(2.7)}\text{ \lparen the equation\rparen} \\ \end{gathered}`

To get the amount remaining after 5 days, we will substitute t with 5

`\begin{gathered} N(t)\text{ = 2\lparen}(1)/(2))^{(5)/(2.7)} \\ \\ N(t)\text{ = 2\lparen}(1)/(2))^(1.8519) \\ N(t)\text{ = 2\lparen0.2770\rparen} \\ N(t)\text{ = 0.554} \end{gathered}`

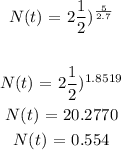
The amount of Au-198 remaining after 5days is 0.554mg