Question

A larger number is 12 more than the smaller number the sum is 74 find the larger number

Answer 1

The larger number is 12 more than the smaller number

`x+12=y`

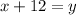
the sum of the two numbers is 74; therefore,

`x+y=74`

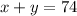
Therefore, we have two equations and two unknowns. We solve them by substitution

Putting in the first into the equation the second gives

`x+(x+12)=74`
`2x+12=74`

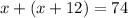
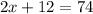
subtracting 12 from both sides gives

`2x=62`

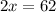
Dividing both sides by 2 gives

`x=31.`

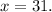
Hence, the the value of the smaller number is 31