Question

If the degree of a polynomial function is odd and the leading coefficient is positive, then the end behavior of the function is___________.

Answer 1

`f(x)\to-\infty\text{ as }x\to-\infty\text{ and }f(x)\to\infty\text{ as }x\to\infty`

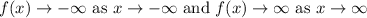
Explanation:

In order to determine the end behaviors of the graphs of polynomials, we use the leading coefficient test.

By the leading coefficient test, when the degree is odd and the leading coefficient is positive, the graph falls to the left and rises to the right.

That is:

`f(x)\to-\infty\text{ as }x\to-\infty\text{ and }f(x)\to\infty\text{ as }x\to\infty`

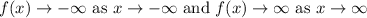
The third option is correct.